
Lim x → a cos x = lim x → a cos ( ( x − a ) + a ) rewrite x = x − a + a = lim x → a ( cos ( x − a ) cos a − sin ( x − a ) sin a ) apply the identity for the cosine of the sum of two angles = cos ( lim x → a ( x − a ) ) cos a − sin ( lim x → a ( x − a ) ) sin a lim x → a ( x − a ) = 0, and sin x and cos x are continuous at 0 = cos ( 0 ) cos a − sin ( 0 ) sin a evaluate cos(0) and sin(0) and simplify = 1 To do this, we must show that lim x → a cos x = cos a lim x → a cos x = cos a for all values of a.

We begin by demonstrating that cos x cos x is continuous at every real number. Trigonometric functions are continuous over their entire domains. If, for example, lim x → a + f ( x ) ≠ f ( a ), lim x → a + f ( x ) ≠ f ( a ), we would need to lift our pencil to jump from f ( a ) f ( a ) to the graph of the rest of the function over ( a, b ]. Requiring that lim x → a + f ( x ) = f ( a ) lim x → a + f ( x ) = f ( a ) and lim x → b − f ( x ) = f ( b ) lim x → b − f ( x ) = f ( b ) ensures that we can trace the graph of the function from the point ( a, f ( a ) ) ( a, f ( a ) ) to the point ( b, f ( b ) ) ( b, f ( b ) ) without lifting the pencil. Continuity over other types of intervals are defined in a similar fashion. Analogously, a function f ( x ) f ( x ) is continuous over an interval of the form ( a, b ] ( a, b ] if it is continuous over ( a, b ) ( a, b ) and is continuous from the left at b. A function f ( x ) f ( x ) is continuous over a closed interval of the form if it is continuous at every point in ( a, b ) ( a, b ) and is continuous from the right at a and is continuous from the left at b. Ī function is continuous over an open interval if it is continuous at every point in the interval. Ī function f ( x ) f ( x ) is said to be continuous from the left at a if lim x → a − f ( x ) = f ( a ). These examples illustrate situations in which each of the conditions for continuity in the definition succeed or fail.Ī function f ( x ) f ( x ) is said to be continuous from the right at a if lim x → a + f ( x ) = f ( a ).
#Calculating continuity calculus how to
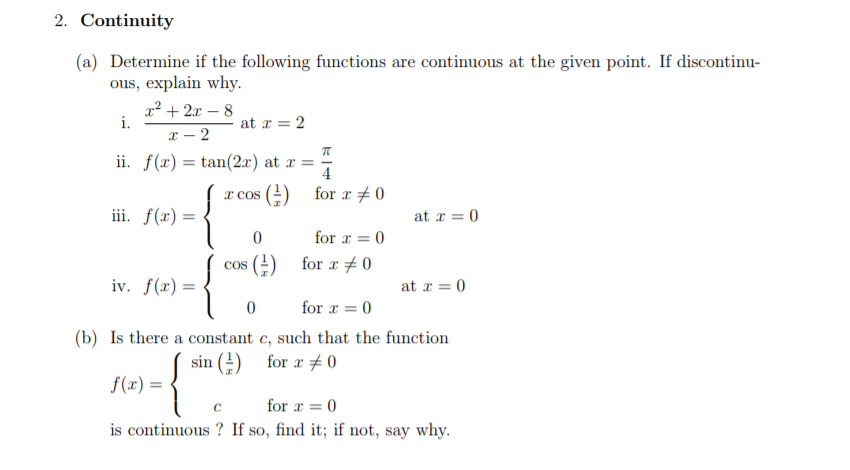
The next three examples demonstrate how to apply this definition to determine whether a function is continuous at a given point. If lim x → a f ( x ) = f ( a ), lim x → a f ( x ) = f ( a ), then the function is continuous at a. If lim x → a f ( x ) ≠ f ( a ), lim x → a f ( x ) ≠ f ( a ), then the function is not continuous at a.
Our first function of interest is shown in Figure 2.32. We then create a list of conditions that prevent such failures. Continuity at a Pointīefore we look at a formal definition of what it means for a function to be continuous at a point, let’s consider various functions that fail to meet our intuitive notion of what it means to be continuous at a point. Intuitively, a function is continuous at a particular point if there is no break in its graph at that point. We begin our investigation of continuity by exploring what it means for a function to have continuity at a point. They are continuous on these intervals and are said to have a discontinuity at a point where a break occurs. Other functions have points at which a break in the graph occurs, but satisfy this property over intervals contained in their domains. Many functions have the property that their graphs can be traced with a pencil without lifting the pencil from the page. 2.4.5 Provide an example of the intermediate value theorem.



 0 kommentar(er)
0 kommentar(er)
